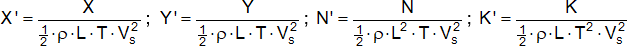Case 3.1: KCS Deep water, forces & moments
CASE 3.1
1 Description of case
- KCS hull shape
- Fixed in surge, sway, roll and yaw. Upright condition (Ø=0). The experimental results are obtained free to trim and sink
- Calm water
- Vmodel=1.0 m/s, Fn = 0.216 (corresponding to full scale 19.9 knots, model scale 1.0 m/s)
- LPP = 2.19 m (scale 105)
- g = 9.81 [m/s2], ρ=1000 [kg/m3]; ν=1.27×10-6 [m2/s]
- Propeller NOT present.
- Rudder present, but at zero rudder angle.
- KG = 0.118m in the model
2 Experimental data
The submissions will be compared to model tests carried out by JMU in September 2012. These model tests were without propeller. The K moment is measured.
The origin is midship. The forces and moments are represented in the body-fixed coordinate.
Reference: Y. Yoshimura, Y. Fukui, H. Yokota and H. Yano: Mathematical Model for Manoeuvring Simulation including Roll motion, Conference Proc. JASNAOE Vol. 16 pp.17-20, 2013.]
1.3 Requested computations
The requested info comes in 4 packages.
- You can only deliver package 2 when you deliver package 1.
- You can only deliver package 3 when you deliver package 2.
- You can only deliver package 4 when you deliver package 3.
Forces and moments should be supplied as follows:
- The N-moment (moment around the z-axis) should be supplied w.r.t. the midship.
- The K-moment (moment around the x-axis) should be supplied w.r.t. the waterline.
- Only the hydrodynamic forces are to be supplied: the inertial forces are not to be included.
- Forces and moments are to be given non-dimensional:
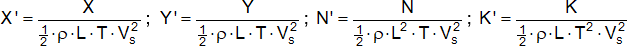
| Package |
Drift angle
(β=-atan(v/u) |
Non-dimensional rate of turn r’ |
Requested quantities |
| Package 1 |
0° |
0 |
X’, Y’, K’, N’ |
| 3° |
0 |
X’, Y’, K’, N’ |
| 6° |
0 |
X’, Y’, K’, N’ |
| 9° |
0 |
X’, Y’, K’, N’ |
| 12° |
0 |
X’, Y’, K’, N’ |
| 18° |
0 |
X’, Y’, K’, N’ |
| Package 2 |
0° |
0.2 |
X’, Y’, K’, N’ |
| 3° |
0.2 |
X’, Y’, K’, N’ |
| 6° |
0.2 |
X’, Y’, K’, N’ |
| 9° |
0.2 |
X’, Y’, K’, N’ |
| 12° |
0.2 |
X’, Y’, K’, N’ |
| 18° |
0.2 |
X’, Y’, K’, N’ |
| Package 3 |
0° |
0.4 |
X’, Y’, K’, N’ |
| 3° |
0.4 |
X’, Y’, K’, N’ |
| 6° |
0.4 |
X’, Y’, K’, N’ |
| 9° |
0.4 |
X’, Y’, K’, N’ |
| 12° |
0.4 |
X’, Y’, K’, N’ |
| 18° |
0.4 |
X’, Y’, K’, N’ |
| Package 4 |
0° |
0.6 |
X’, Y’, K’, N’ |
| 3° |
0.6 |
X’, Y’, K’, N’ |
| 6° |
0.6 |
X’, Y’, K’, N’ |
| 9° |
0.6 |
X’, Y’, K’, N’ |
| 12° |
0.6 |
X’, Y’, K’, N’ |
| 18° |
0.6 |
X’, Y’, K’, N’ |
1.4 Format
Link to an excel file, which a submitter can download.
The forces and moments in the excel sheet should be filled in. The excel sheet should be send to the organizing committee per email address “
simman2019host@gmail.com”.
5 Example
For KCS, we elaborated an actual example of an actual submission compared to actual experimental data.
Table 3 gives an example of the data that we would be getting for the KCS for case 3.1.1 and 3.1.2. Submitters should generate an excel sheet in the format of Table 3. A template excel sheet will be supplied on the website. The submitter has to type the calculated X’, Y’ N’ and K’ for the hull of the KCS.
The table below gives the results for the KCS in upright condition (Ø=0). The speed is 19.9 knots, the rudder angles remain zero. The N-moment is to be calculated w.r.t. midship. The K-moment is to be calculated w.r.t. the still water waterline. The forces and moments for this case (KCS measured by JMU) should include the rudder at zero angle, but no propeller.
Table: Data from “submission 1”, in this case of KCS (this is an actual case, where an empirical method is used to generate the forces and moments for the state).
|
β(deg)=atan(-v/u) |
r’ |
X’ |
Y’ |
N’ |
K’ |
| Case3.1 |
20 |
0 |
-1.2473E-02 |
1.7368E-01 |
4.3696E-02 |
-8.6842E-02 |
| 16 |
0 |
-1.3579E-02 |
1.1751E-01 |
3.0475E-02 |
-5.8754E-02 |
| 12 |
0 |
-1.4356E-02 |
7.3700E-01 |
2.0212E-02 |
-3.6850E-02 |
| 8 |
0 |
-1.4851E-02 |
4.1024E-01 |
1.2369E-02 |
-2.0512E-02 |
| 4 |
0 |
-1.5120E-02 |
1.7368E-01 |
6.0277E-03 |
-8.6842E-03 |
| 0 |
0 |
-1.5204E-02 |
0.0000E+00 |
0.0000E+00 |
0.0000E+00 |
| Case3.2 |
20 |
0.2 |
-1.8348E-02 |
1.3451E-01 |
2.1019E-02 |
-6.7257E-02 |
| 16 |
0.2 |
-1.8348E-02 |
8.7615E-02 |
1.3691E-02 |
-4.3808E-02 |
| 12 |
0.2 |
-1.8258E-02 |
4.9219E-02 |
7.5291E-03 |
-2.4610E-02 |
| 8 |
0.2 |
-1.7607E-02 |
1.8811E-02 |
2.2856E-03 |
-9.4057E-03 |
| 4 |
0.2 |
-1.6636E-02 |
-4.7776E-03 |
-2.3528E-03 |
2.2888E-03 |
| 0 |
0.2 |
-1.5410E-02 |
-2.6181E-02 |
-8.2461E-03 |
1.3091E-02 |
| Case3.3 |
20 |
0.4 |
-2.4079E-02 |
1.0617E-01 |
7.3050E-04 |
-5.3084E-02 |
| 16 |
0.4 |
-2.4079E-02 |
6.3392E-02 |
-2.4201E-03 |
-3.1696E-02 |
| 12 |
0.4 |
-2.2190E-02 |
2.8965E-02 |
-5.2659E-03 |
-1.4482E-02 |
| 8 |
0.4 |
-2.0519E-02 |
-1.4328E-04 |
-8.2461E-03 |
7.1638E-05 |
| 4 |
0.4 |
-1.8424E-02 |
-2.8655E-02 |
-1.3422E-02 |
1.4328E-02 |
| 0 |
0.4 |
-1.5977E-02 |
-5.8238E-02 |
-2.1176E-02 |
2.9119E-02 |
| Case3.4 |
20 |
0.6 |
-2.9594E-02 |
8.9161E-02 |
-1.7859E-02 |
-4.4581E-02 |
| 16 |
0.6 |
-2.8118E-02 |
4.9734E-02 |
-1.7478E-02 |
-2.4867E-02 |
| 12 |
0.6 |
-2.6055E-02 |
1.4225E-02 |
-1.8330E-02 |
-7.1123E-03 |
| 8 |
0.6 |
-2.3457E-02 |
-2.0615E-02 |
-2.2027E-02 |
1.0308E-02 |
| 4 |
0.6 |
-2.0321E-02 |
-5.7208E-02 |
-2.8458E-02 |
2.8604E-02 |
| 0 |
0.6 |
-1.6781E-02 |
-9.4830E-02 |
-3.7869E-02 |
4.7415E-02 |
The results are made non-dimensional using the following formulae:

Using the example of Table 3, the organisers will compare these results to experimental data. Comparing submission 1 (Table 3) to experimental data will give Figure 1. It is the intention to have many submissions in the same type of figure to learn about the prediction capabilities for which combinations of r’ and β.
 Figure 1: Comparison of submission 1 to the experimental data for KCS
Figure 1: Comparison of submission 1 to the experimental data for KCS